This lesson will cover a few examples to illustrate the equation of the tangent to a circle in point form. Let’s begin.
Example 1 Find the equation of the tangent to the circle x2 + y2 = 25, at the point (4, -3)
Solution Note that the problem asks you to find the equation of the tangent at a given point, unlike in a previous situation, where we found the tangents of a given slope.
Therefore, we’ll use the point form of the equation from the previous lesson.
The required equation will be x(4) + y(-3) = 25, or 4x – 3y = 25.
Example 2 Find the equation of the tangent to the circle x2 + y2 – 2x – 6y – 15 = 0 at the point (5, 6).
Solution This one is similar to the previous problem, but applied to the general equation of the circle.
We’ll use the point form once again. The required equation will be x(5) + y(6) + (–2)(x + 5) + (– 3)(y + 6) – 15 = 0, or 4x + 3y = 38.
Example 3 Find the point where the line 3x + 4y = 25 touches the circle x2 + y2 = 25
Solution We’ve done a similar problem in a previous lesson, where we used the slope form. Here, I’m interested to show you an alternate method.
The problem has given us the equation of the tangent: 3x + 4y = 25.
But we know that any tangent to the given circle looks like xx1 + yy1 = 25 (the point form), where (x1, y1) is the point of contact.
Therefore, to find the values of x1 and y1, we must ‘compare’ the given equation with the equation in the point form. Head over to this lesson, to understand what I mean about ‘comparing’ lines (or equations).
On comparing the coefficients, we get x1/3 = y1/4 = 25/25, which gives the values of x1 and y1 as 3 and 4 respectively.
The point of contact therefore is (3, 4).
Example 4 Find the point where the line 4y – 3x = 20 touches the circle x2 + y2 – 6x – 2y – 15 = 0.
Solution This problem is similar to the previous one, except that now we don’t have the standard equation.
We’ll use the new method again – to find the point of contact, we’ll simply compare the given equation with the equation in point form, and solve for x1 and y1.
The equation of the tangent in the point for will be xx1 + yy1 – 3(x + x1) – (y + y1) – 15 = 0, or x(x1 – 3) + y(y1 – 1) = 3x1 + y1 + 15.
On comparing the coefficients, we get (x1 – 3)/(-3) = (y1 – 1)/4 = (3x1 + y1 + 15)/20. On solving the equations, we get x1 = 0 and y1 = 5.
Therefore, the point of contact will be (0, 5).
Note that in the previous two problems, we’ve assumed that the given lines are tangents to the circles. Comparing non-tangents to the point form will lead to some strange results, which I’ll talk about sometime later.
One more.
Example 5 Show that the tangent to the circle x2 + y2 = 25 at the point (3, 4) touches the circle x2 + y2 – 18x – 4y + 81 = 0. Also find the point of contact.
Solution The following figure (inaccurately) shows the complicated situation:
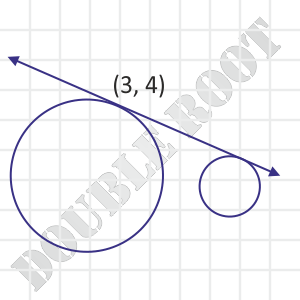
The problem has three parts – finding the equation of the tangent, showing that it touches the other circle and finally finding the point of contact.
We’ve got quite a task ahead, let’s begin!
The equation can be found using the point form: 3x + 4y = 25
To prove that this line touches the second circle, we’ll use the condition of tangency, i.e. its distance from the center of the circle must be equal to its radius.
The circle’s center is (9, 2) and its radius is 2.
The distance of the line 3x + 4y – 25 = 0 from (9, 2) is |3(9) + 4(2) – 25|/5 = 2, which is equal to the radius. Almost done!
Now to find the point of contact, I’ll show yet another method, which I had hinted in a previous lesson – it’ll be the foot of perpendicular from the center to the tangent.
To find the foot of perpendicular from the center, all we have to do is find the point of intersection of the tangent with the line perpendicular to it and passing through the center.
The required perpendicular line will be (y – 2) = (4/3)(x – 9) or 4x – 3y = 30.
And the final step – solving the obtained line with the tangent gives us the foot of perpendicular, or the point of contact as (39/5, 2/5).
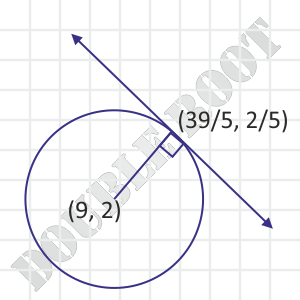
Phew! We’re finally done. That’ll be all for this lesson.
The next lesson cover tangents drawn from an external point.