Tangent: Slope Form (Part 1)

This lesson will talk about a special case of intersection of a line with a circle – the case where the line touches the circle.

Suppose we have a circle x² + y² = a² and a line y = mx + c. For what relation between a, m, and c will the given line touch (i.e. become a tangent to) the circle?

As discussed in the previous lessons, we can understand this in two different ways – one using quadratic equations, and the other using geometry.

Let’s try the first one.

Method 1

On solving the equation of the line with that of the circle, we get the same quadratic as before:

(1 + m²)x² + 2cmx + c² – a²_­ = 0

Recall that the roots of this equation represent nothing but the x-coordinates of the points of intersection of the two curves. If the line touches the circle, then the roots of this quadratic equation must be equal, or coincident.

Let’s do the calculations then!

Now,

D = 4c²m² – 4(c² – a²)(1 + m²)

Putting this equal to zero, we get

c² = a²(1 + m²)

⇒ c = ±a\(\sqrt{1+m^2}\)

And that’s it. We’ve obtained the required condition.

This means that the line y = mx + c will touch the circle x² + y² = a² if:

c = ±a\(\sqrt{1+m^2}\)

In other words, the line y = mx ± a\(\sqrt{1+m^2}\), will touch the circle x² + y² = a² for all values of m.

Notice that we get two different values of c, for a given m, which means that we’ll get two different tangents of a given slope. Here’s a figure that shows the two (parallel) tangents.

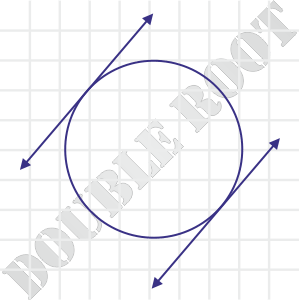
Let’s try the second method, which uses geometry.

Method 2

The line y = mx + c will touch the given circle if its distance from the center of the circle equals the radius of the circle.

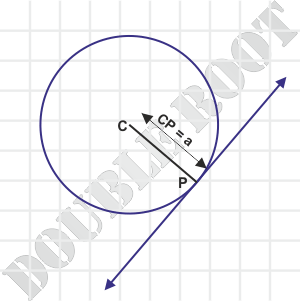
The center of the circle is (0, 0) and the radius is a. On applying the above condition, we get

|m(0) – (0) + c|/\(\sqrt{1+m^2}\) = a

⇒ |c| = a\(\sqrt{1+m^2}\)

We got the same condition as obtained before. But we’ll always prefer this method over the previous one, as it involves fewer calculations.

Lesson Summary

1. For the circle x² + y² = a², the equation of the tangent whose slope is ‘m’, is given by y = mx ± a\(\sqrt{1+m^2}\)
   This equation is referred to as the ‘slope form’ of the tangent. Given the slope, we can obtain the equation of the tangent
2. The condition for a given line to touch a circle is: Distance of the line from the center of the circle, must be equal to its radius. We’ll refer this as the ‘condition of tangency’.

In other cases, we might be given a point on the circle, at which a tangent can be drawn. Or, we might be given the point outside the circle, from which two tangents can be drawn to the circle. To obtain the tangents in these situations, you’ll have to wait for a few more lessons!

A few things have been left unsaid in this lesson – the equation of the tangent in slope form to the circle whose center is not at origin, and the point where the tangent will touch the circle. I’ll talk about this in the next lesson.