Tangent from an external point (Part 2)

This lesson will discuss how to find the angle between the tangents drawn from an external point. There’ll be two methods again, one will use the slope form of the tangent, and the other using geometrical conditions (valid only in case of the circle). Let’s begin.

Method 1

The circle we’ll use will be x² + y² = a² again. We’re interested to find the angle between the tangents drawn from an external point, say (x₁, y₁).

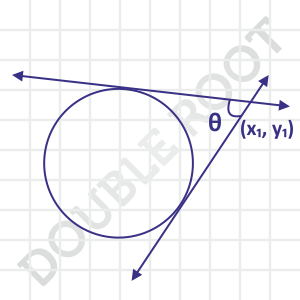
The tangent to this circle will be y = mx + a\(\sqrt{1+m^2}\)  (slope form). And since it is drawn from the point (x₁, y₁), the coordinates must satisfy the equation of the tangent, that is y₁ = mx₁ + a \(\sqrt{1+m^2}\) , which is equivalent to (y₁ – mx₁)² = a²(1 + m²)

Similar to the previous lesson, solving this quadratic will give us the values of m, say m₁ and m₂, and using the formula for angle between two lines, we’re done.

But we can do better, we do not need to find the values of m₁ and m­₂. Have a look at the formula again: tanθ = |m₁ – m₂|/|1 + m₁m₂|

All we need to do is find the difference of the roots and their product, and plug them in the above formula. (I suggest you visit this link for the formulas for product and difference of the roots)

Method 2

As mentioned earlier, things (sometimes) get easy when dealing with circles, because of their geometrical properties.

Have a look at the following figure again:

Now we need to find that angle (θ) by using geometry. Turns out its quite easy to do so!

I’ll join that external point with the center of the circle, and also join the centre with one of the points of contact. I’ll also give the points some names – P, Q and C. This is what we get:

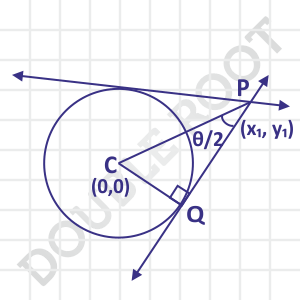
As you might have guessed, the angle θ will be divided into half, due to symmetry (This can also be proved using congruence of triangles).

Now here’s the magic: The distance CQ is equal to the circle’s radius. And CP can easily be found using the distance formula. And using a little trigonometry, we have sin(θ/2) = CQ/CP, which will give us the value of θ. And we’re done!

We’ll prefer the second method to the first one, due to its simplicity. But the first one will be our only option when we deal with parabolas, ellipses and hyperbolas.

Lesson Summary

The angle (θ) between tangents from an external point to a circle can be found using the following two methods:

1. tanθ = |m₁ – m₂|/|1 + m₁m₂|, where |m­₁ – m_2­| and m₁m_2­ will be found out from the quadratic equation obtained by substituting the coordinates of the given point the slope form of the tangent.
2. If P be the external point and C be the center of the circle, then sin(θ/2) = r/CP, where r is the radius of the circle.

That’ll be all for this lesson. In case you’re confused, head over to this lesson for some related examples.

The next lesson will cover another simple concept – length of a tangent from an external point. See you there !