Hello. This lesson will cover another simple concept – finding out the length of the tangent to a circle, drawn from an external point.
Let’s say that the tangent is drawn to the circle x2 + y2 = a2, from the point (x1, y1).
A figure to illustrate:
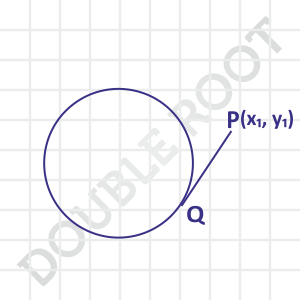
With reference to the figure above, we need to find the length PQ.
Now, one way to reach our answer is to find the coordinates of Q, the point of contact, and use the distance formula to find PQ.
But finding the point of contact is a rather lengthy process, which I’ll avoid. We can do much better, using geometry. Have a look at the following figure.
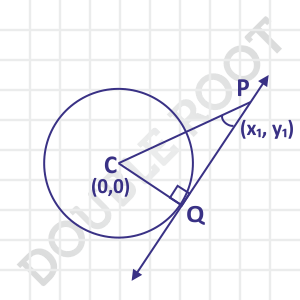
The distance CQ is equal to the circle’s radius, and CP can be found using the distance formula. Finally, we can use the Pythagoras’ Theorem to find PQ, i.e. the length of the tangent. Let’s do it!
CQ = a, and CP = \( \sqrt{{x_1}^2 +{y_1}^2} \)
Therefore, PQ = \( \sqrt{CP^2-CQ^2} = \sqrt{{x_1}^2 +{y_1}^2 – a^2}\)
Things don’t end here.
Just to show you a pattern, let’s consider the general equation: x2 + y2 + 2gx + 2fy + c = 0
In this case CQ or the radius will be \( \sqrt{g^2 + f^2 – c}\) . And CP = \( \sqrt{(x_1 + g)^2 + (y_1 + f)^2}\)
By using the Pythagoras’ theorem again, we obtain PQ as \( \sqrt{{x_1}^2 + {y_1}^2+ 2gx_1 + 2fy_1 + c} \)
Do you see it?
The lengths of the tangents obtained are \( \sqrt{{x_1}^2 +{y_1}^2 – a^2}\) and \( \sqrt{{x_1}^2 + {y_1}^2 + 2gx_1 + 2fy_1 + c} \) respectively in the cases when the equations were x2 + y2 – a2 = 0 and x2 + y2 + 2gx + 2fy + c = 0.
That is, the length of the tangent is obtained by substituting the coordinates of the point in the circle’s equation and taking the square root of the value obtained. Pretty neat!
Like me, you might be wondering what’ll happen when the quantity x12 + y12 – a2 turns out to be zero, or even worse, negative. Aren’t you?
Well, if you are, let’s rewind back to earlier times, when we learnt about position of a point with respect to a circle. The expression x12 + y12 – a2 told us whether the point (x1, y1) lied outside, on or inside the circle x2 + y2 – a2 = 0.
If it is positive, the point lies outside the circle, and we can take it’s square root to obtain the (positive) length of the tangent drawn from the point.
If it is zero, that means the point lies on the circle and we get a tangent of length zero.
And if it’s negative, the point lies inside the circle. We cannot find the length of the tangent by taking its square root, because we cannot draw a tangent to the circle from a point lying inside it.
So, it’s all connected, and makes a lot of sense! That’s what I love about coordinate geometry. Hope that one day you feel the same too.
Lesson Summary
- The length of the tangent from the point (x1, y1) to the circle x2 + y2 – a2 = 0 is \( \sqrt{{x_1}^2 +{y_1}^2 – a^2}\)
- The length of the tangent from the point (x1, y1) to the circle x2 + y2 + 2gx + 2fy + c = 0 is \( \sqrt{{x_1}^2 + {y_1}^2 + 2gx_1 + 2fy_1 + c} \)
I’ll end this lesson here, and the next one will cover a few examples to illustrate the concept covered in this and the last two lessons. See you there!