Problem 1
ABCD is a quadrilateral and E, F, G, and H are the midpoints of its sides AB, BC, CD, and DA respectively. If P be any point inside the quadrilateral, then prove that:
ar(AEPH) + ar(PFCG) = ar(EBFP) + ar(PGDH)
Here’s a simulation that demonstrates what needs to be proven.
You can drag P and the the four vertices and observe the two sums of the areas.
Solution
I won’t provide the full solution here, but only give you some tools and hints that’ll help you solve the problem yourself.
Notice that we do not need to find the values of the areas. In fact, we cannot. Because, none of the angle or side measures are given, and P is also a variable point. Instead, we need to focus on the sums of these areas.
We could still choose to assume some variables and find the areas in terms of these, but that could create a mess.
So, midpoints – that’s the only extra information we have here. Reminds me of medians, and medians are related to areas. Here’s how.
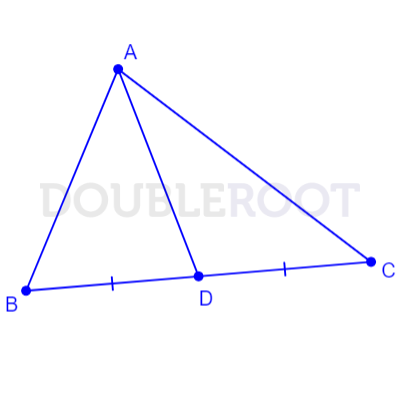
In the above triangle, AD is the median, i.e., BD = DC. Then,
ar(ABD) = ar(ACD)
Why? Because, if h be the length of the altitude from A, then
ar(ABD) = 1/2 x BD x h,
ar(ACD) = 1/2 x DC x h, and
BD = DC
But to use this property, we need triangles. And there are none, in the original figure.
Now, I suggest you proceed further on your own from this point.
Were you able to solve it using the above hints? Or, did you solve using any other method? Or, do you need some more help? You can reach out at the Facebook page.
Problem Source: GoGeometry
Further Research
What if the point P lies outside the quadrilateral? Will get any special results? Try dragging P outside ABCD in the simulation above to see if you get anything. Then, see if you can prove that as well!