Welcome to Lesson 5. Before I proceed toward examples, I’ll cover some basic but useful applications of section formula. Here they are !
Midpoint of a line segment
Hmm.. how do we relate section formula with midpoint? Simple! The midpoint divides the line joining the two points in the ratio 1:1 (internally).
Consider the points to be P(x1, y1) and Q(x2, y2) and let R(x, y) be the midpoint of PQ. Using the formula for internal division, we have x =\( \large \frac{1.x_2+1.x_1}{1+1} = \frac{x_1+x_2}{2}\) and y=\( \large \frac{1.y_2+1.y_1}{1+1} = \frac{y_1+y_2}{2}\) The expression for midpoint is quite useful, and you’ll have to remember this as a formula. And likewise with the following ones.
Centroid of a triangle
Recall that the centroid is the point of concurrency of the medians, and a median is a line joining the vertex to the midpoint of the opposite side.
So how do we get the centroid? The centroid of a triangle divides each of the medians in the ratio 2:1. I’ll illustrate this by a figure.
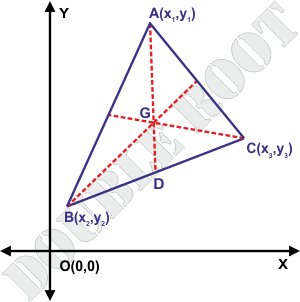
Consider a triangle having vertices as A(x1, y1), B(x2, y2) and C(x3, y3) whose medians intersect at G(x, y). Here BD = DC, and AG:GD = 2:1. So all we have to do is find the coordinates of D, followed by G, using section formula.
The coordinates of D will be \( \large (\frac{x_2+x_3}{2}, \frac{y_2+y_3}{2})\) (midpoint of BC) Now, the centroid G divides AD in the ratio 2 : 1, so the coordinates of G will be x=\( \large \frac{2\frac{x_2+x_3}{2}+1.x_1}{2+1}=\frac{x_1+x_2+x_3}{3}\) and \( \large y=\frac{2\frac{y_2+y_3}{2}+1.y_1}{2+1}=\frac{y_1+y_2+y_3}{3}\)
Moving on..
Incentre of a triangle
The incentre is the point of concurrency of the internal angle bisectors of a triangle. Consider a triangle having vertices as A(x1 , y1 ), B(x2 , y2 ) and C(x3 , y3 ) whose internal angle bisectors intersect at I(x, y).
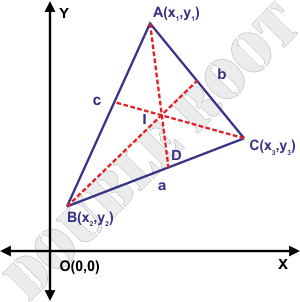
The section formula has a role here too ! If AD is the internal angle bisector of angle A, meeting the opposite side BC in D, then AB/AC = BD/DC (Angle Bisector Theorem) That is, D divides BC in the ratio c : b internally. (I’ve labelled the sides lengths a, b, and c for simplicity. The values can be found out using distance formula.)
We have the coordinates of D which are \( \large (\frac{bx_2+cx_3}{b+c},\frac{by_2+cy_3}{b+c})\) What now?
Consider the triangle ABD. We have BI as the bisector of angle B. We can use the angle bisector theorem once again to write BD/BA = DI/IA. That means, I divides DA in the ratio BD:BA.
Now if only we could find BD… can we? Of course we can !
The point D divides BD in the ratio c:b, which makes BD equal to \( \large \frac{ca}{c+b}\).
Therefore, BD/BA = \( \large \frac{a}{b+c}\) = DI/IA, or I divides DA in the ratio a : b+c.
Now using section formula again, we have the coordinates of I as \( \large (\frac{ax_1+bx_2+cx_3}{a+b+c},\frac{ay_1+by_2+cy_3}{a+b+c}) \)
Phew ! That was tiring.. ! I’ll wind up with the excentre.
Excentre of a triangle
The excentre is the point of concurrency of two external angle bisectors and one internal angle bisector of a triangle.
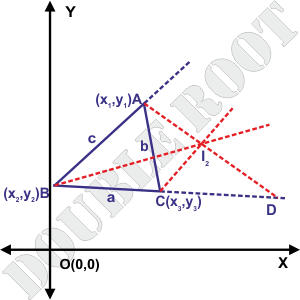
Hmm.. that looks a bit complicated. It isn’t !
The same angle bisector theorem applies here as well. AB/AC is still equal to BD/DC. Or, BD/DC=c/b. Or, D divides BC in the ratio c:b, but externally – this is the only difference !
I’m going to skip all the calculations and write the coordinates of I2 \( \large (\frac{ax_1-bx_2+cx_3}{a-b+c},\frac{ay_1-by_2+cy_3}{a-b+c})\) (the ‘2’ denotes the excentre opposite to B(x2, y2). There are three such excentres)
Please make sure you are able to derive the above result yourself by proceeding in the same way as deriving the incentre’s coordinates.
The coordinates of the other two excentres are I1\( \large (\frac{-ax_1+bx_2+cx_3}{-a+b+c},\frac{-ay_1+by_2+cy_3}{-a+b+c})\) I3\( \large (\frac{ax_1+bx_2-cx_3}{a+b-c},\frac{ay_1+by_2-cy_3}{a+b-c})\)
Lesson Summary
- The midpoint of the line segment joining (x1, y1) and (x2, y2) is given by \( \large \large \left ( \frac{x_1+x_2}{2} , \frac{y_1+y_2}{2} \right ) \)
- The centroid of a triangle whose vertices are (x1, y1), (x2, y2) and (x3, y3) is given by \( \large \left ( \frac{x_1+x_2+x_3}{3} , \frac{y_1+y_2+y_3}{3} \right ) \)
- The incenter of a triangle whose vertices are (x1, y1), (x2, y2) and (x3, y3) is given by \( \large \large \left ( \frac{ax_1+bx_2+cx_3}{a+b+c} , \frac{ay_1+by_2+cy_3}{a+b+c} \right ) \), where a, b and c are the lengths of the sides opposite the vertices (x1, y1), (x2, y2) and (x3, y3) respectively.
- The excenters of a triangle whose vertices are (x1, y1), (x2, y2) and (x3, y3) are given by \( \large \large \left ( \frac{-ax_1+bx_2+cx_3}{-a+b+c} , \frac{-ay_1+by_2+cy_3}{-a+b+c} \right ) \), \( \large \large \left ( \frac{ax_1-bx_2+cx_3}{a-b+c} , \frac{ay_1-by_2+cy_3}{a-b+c} \right ) \) and \( \large \large \left ( \frac{ax_1+bx_2-cx_3}{a+b-c} , \frac{ay_1+by_2-cy_3}{a+b-c} \right ) \), respectively opposite the vertices (x1, y1), (x2, y2) and (x3, y3).
That’s it for now, see you in the next lesson with a few examples.
\(\)