Welcome to the second part of the lesson. I’ll be covering the remaining examples (covered here) in this part.
The first one was PA+PB=AB. Let A be (0, 0) and B be (4, 4) and the moving point P be (x, y). All we have to do here is write the given relation in terms of equations and formulas.
Now, PA\(=\sqrt{(x-0)^2+(y-0)^2}\), PB\(=\sqrt{(x-4)^2+(y-4)^2}\) and AB\(=\sqrt{(4-0)^2+(4-0)^2}\)
So the given relation becomes
\(\sqrt{x^2+y^2}+\sqrt{(x-4)^2+(y-4)^2}=\sqrt{4^2+4^2}\) or \(\sqrt{(x-4)^2+(y-4)^2}=\sqrt{32}-\sqrt{x^2+y^2}\).
This can be simplified by doing some squaring and cancelling (please try it out on your own)
The final equation will be x – y = 0. Here’s a figure to illustrate
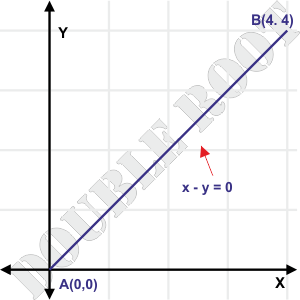
(Note that the line will not extend beyond A and B)
To the last example !
To remind, the condition here was the point P should move (in a plane) such that angle APB is always 90 degrees, where A and B are fixed points.
Let A be (0, 0) and B be (2, 0), and P be (x, y). Now how can we write the given condition in mathematical form?
Think about it… any idea? Our old friend Pythagoras! AP2 + BP2 must be equal to AB2
That is [x2 + y2]+[(x – 2)2 + y2] = (2 – 0)2 + (0 – 0)2 or x2 + y2 – 2x = 0.
For the homework I gave (here), the locus will an arc of a circle, whose chord AB subtends an angle of 60o on the arc. In fact, it will be two arcs, as shown in the figure below.
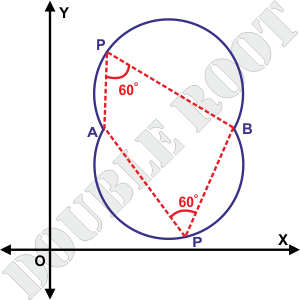
Converting this to an equation will be a little complicated as of now. I’ll leave that for later.
We’re now through with some basic methods of finding out the loci and their equations, under different conditions. In the next lesson, I’ll illustrate some more methods, and cover a few examples.
See you there !