Tangent: Point Form

Hello! This lesson will discuss the equations of a tangent to a circle at a given point.

Equation of a Tangent

Let’s consider the standard circle first: x² + y² = a². We need to find the equation of the tangent to this circle at a given point, say (x₁, y₁). Have a look at the following figure to understand what I’m talking about.

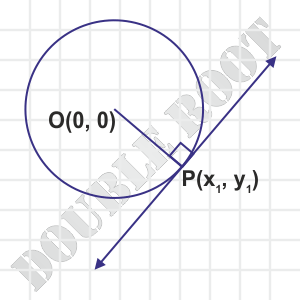
Let’s find it’s equation now.

We know that the line passes through the point (x₁, y₁). Now if only we could find it’s slope…

Turns out we can! We’ll use the fact that the line joining the center of the circle to the point of contact is perpendicular to the tangent.

The slope of OP will be y₁/x₁. Using what we learnt in straight lines, the slope of the tangent will be –x₁/y₁.

And that’s it! The required equation, using the two point form, will be (y – y₁) = –(x₁/y₁)(x – x₁), which, on rearrangement of terms, looks like this: xx₁ + yy₁ – x₁² – y₁­² = 0.

We can make it look a bit nicer. Since the point (x₁, y₁) lies on the circle, it’s coordinates must satisfy the circle’s equation, or x₁² + y₁­² must equal a².

The equation of the tangent will therefore look like xx₁ + yy₁ – a² = 0. Neat!

And what if the circle is not standard? The method remains exactly the same – except that slope of the tangent will now be –(x₁ + g)/(y₁ + f). A figure for your reference:

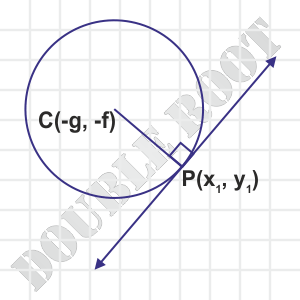
Using the two point form again, doing the rearrangements and some black magic, we’ll get the final equation as xx₁ + yy₁ + g(x + x₁) + f(y + y₁) + c = 0.

And that’ll be all for this lesson.

Lesson Summary

1. The equation of the tangent to the circle x² + y² = a² at the point (x₁, y₁) is xx₁ + yy₁ = a²
2. The equation of the tangent to the circle x² + y² + 2gx + 2fy + c = 0 at the point (x₁, y₁) is xx₁ + yy₁ + g(x + x₁) + f(y + y₁) + c = 0.

The next lesson will cover a few related examples.