This lesson will cover a few examples relating to equations of common tangents to two given circles.
Example 1 Find the equation of the common tangents to the circles x2 + y2 – 2x – 4y + 4 = 0 and x2 + y2 + 4x – 2y + 1 = 0.
Solution These circles lie completely outside each other (go back here to find out why). That means, there’ll be four common tangents, as discussed previously.
We’ll first need to find the points where the direct and the transverse common tangents meet.
As discussed in the previous lesson, these points divide the line joining the centre in the ratio of the radii (internally and externally).
Let’s begin the calculations then. Here,
C1 ≡ (1, 2) and r1 = 1
C2 ≡ (-2, 1) and r2 = 2
Using the section formula, we get the point of intersection of the direct common tangents as (4, 3) and that of the transverse common tangents as (0, 5/3).
Next, we’ll use our knowledge of finding equation of tangents from an external point.
Any line through (4, 3) is
y – 3 = m(x – 4)
⇒ mx – y – 4m + 3 = 0
Now, this line will touch the first (also the second) circle if its distance from the circle’s centre will be equal to its radius. That is,
|m(1) – 2 – 4m + 3|/ \( \sqrt{m^2+1} \) = 1
This gives m = 0 and m = 3/4.
Finally, using the point-slope form, the equations of the direct common tangents will be
y = 4
3x – 4y = 0
Now, let’s find the equations of the transverse common tangents.
Any line through (0, 5/3) is
y – 5/3 = m(x – 0)
⇒ 3mx – 3y + 5 = 0
Doing the same magic again, we get
|3m(1) – 3(2) + 5|/ \( \sqrt{9m^2 + 9} \) = 1
This gives m = -4/3 and m = ∞, giving the equation of the tangents as
x = 0
4x + 3y = 5
Wait, where did that ∞ come from? I’m not explaining that at the moment – maybe when I cover quadratic equations someday. You can search for ‘quadratic equation with roots as infinity’ to gain some knowledge about that.
Finally, here’s a figure showing it all.
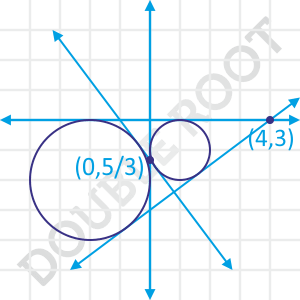
It’s a long process, but you’ll get fast at it after enough practice.
Example 2 Find the equation of the common tangents to the circles x2 + y2 – 6x = 0 and x2 + y2 + 2x = 0.
Solution These circles touch externally, which means there’ll be three common tangents.
I won’t be deriving the direct common tangents’ equations here, as the method is exactly the same as in the previous example.
Instead, I’m interested in the third one, which touches them at their point of contact. This point is the one dividing the line joining their centres in the ratio of their radii internally.
Here,
C1 ≡ (3, 0) and r1 = 3
C2 ≡ (-1, 0) and r2 = 1
Using the section formula, we get the point of contact as (0, 0).
Now as discussed earlier, the required tangent will be perpendicular to C1C2, and passing through this point. We’ll get the equation of the line as x = 0.
Here’s a picture, worth the previous 100 words, showing what’s happening.
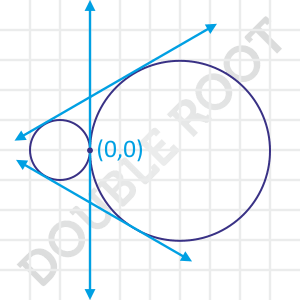
I also suggest you find the other two equations of the tangents. In case you’re interested, their equations are
\(\sqrt{3}\)y = ± (x + 3)
One last example.
Example 3 Find the equation of the common tangents to the circles x2 + y2 = 16 and x2 + y2 – 6x + 8 = 0.
Solution These circles touch internally, which means there’ll be only one common tangent. We’ll first find the point of contact, which divides the line joining the centres in the ratio of the radii externally.
Here,
C1 ≡ (0, 0) and r1 = 4
C2 ≡ (3, 0) and r2 = 1
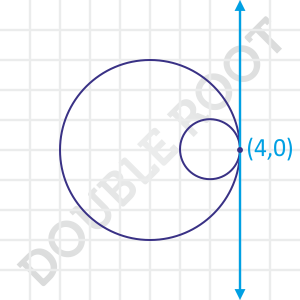
Using the section formula, we get the point of contact as (4, 0).
Once again, the required tangent will be perpendicular to C1C2 and passing through this point. We’ll get the equation of the common tangent as
x = 4
Here’s one last figure showing everything.
And that’ll be all about common tangents. I’ve skipped some calculations here and there, and I suggest you do them yourself. Head back to the straight line lessons in case you’ve forgotten some of the concepts.
Next, I’ll move onto something known as radical axis of two circles.