Problem 9
ABCD is a square and P is a point inside it such that PA = 1, PB = 2, and PC = 3.
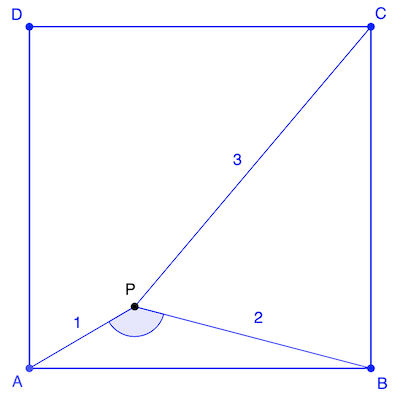
Find the measure of ∠APB.
Solution
To solve this problem, you’ll need to know four things.
Pythagoras Theorem
I’m assuming that you know this one. Anyways, here’s a quick recap:
In a right triangle, the square of the hypotenuse equals the sum of the squares of its other two sides.
Conversely:
If the square of one side of a triangle equals the sum of the squares of its other two sides, then this triangle must be right-angled.
Here’s a little simulation that demonstrates that.
You can drag the three vertices of the right triangle. Is PQ2 always equal to QR2 + RP2?
Angle Sum Property of a Triangle
I’m assuming that you know this one too. Anyways, another quick recap:
The sum of the three angles of a triangles is always 180°.
Another simulation for you.
You can drag the three vertices of the triangle. Is the sum of the three angles always 180°?
Isosceles Triangles
The angles opposite to the equal sides of an isosceles triangle are equal. Conversely, if two angles of a triangle are equal, then the sides opposite to those angles are also equal.
Here’s a simulation that demonstrates that.
You can drag the vertices B and C. Is ∠A always equal to ∠B?
Now, you must be wondering that there are no right triangles to which you can apply Pythagoras’ theorem. Nor there are any isosceles triangles. That brings me to the last thing that you’ll need here.
Black Magic
To proceed, try flipping or rotating the square and align one of the rotated square’s sides with one of the original square’s.
Can you now spot the right angled triangle? What about the isosceles triangle? You might need to join a couple of points. The triangles would [magically] appear!
That’s all I have to say for now. I suggest you proceed on your own from this point. Good luck!
Source: CutTheKnot
Previous Problem | Next Problem | Geometry Problems | Problems Home