9. Two-Point Form

This lesson will cover the equation of the line, which passes through two given points, say (x₁, y₁) and (x₂, y₂). We call this the two-point form of the equation of a line.

We’ll derive the equation using the point-slope form of the equation.

Let’s assume the slope of the line to be m. Then, since the line passes through the point (x₁, y₁), it’s equation will be:

y – y₁ = m(x – x₁) … I

But we do not know the value of m. Instead, we’ve been given another point (x₂, y₂), through which the line passes.

That means, the coordinates (x₂, y₂) must satisfy the above equation. We’ll get:

y₂ – y₁ = m(x₂ – x₁)

⇒ m = (y₂ – y₁)/(x₂ – x₁)

And that’s it – we’ve found the slope. On substituting this in equation (I), we’ll get the required equation as

y – y₁ = {(y₂ – y₁)/(x₂ – x₁)}(x – x₁)

This also tells me one more thing – the slope of the line joining two points, (x₁, y₁) and (x₂, y₂) equals:

(y₂ – y₁)/(x₂ – x₁)

Have a look.

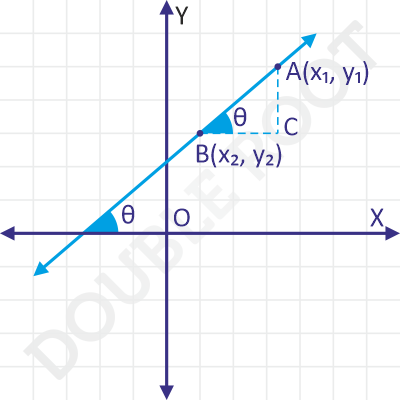
The slope of the line AB equals tanθ or AC/BC or (y₂ – y₁)/(x₂ – x₁). This expression will be quite useful as we’ll see later.

The second method (i.e. starting from scratch) is left for you as an exercise.

Here’s a simulation where you can observe the two-point form of the equation of a line.

You can drag the two points and see how the equation changes. Also, try substituting these coordinates in the equation that we derived. Do you get the same equation as shown in the simulation?

Lesson Summary

1. The equation of the line joining two points (x₁, y₁) and (x₂, y₂) is given by y – y₁={(y₂ – y₁)/(x₂ – x₁)}(x – x₁).
2. The slope of the line joining two points (x₁, y₁) and (x₂, y₂) equals (y₂ – y₁)/(x₂ – x₁).

In the next lesson, I’ll cover a few examples on the two-point form. See you there!