This lesson will discuss a few examples on the slope-intercept form of the equation of a line.
Example 1 Find the equation of the line whose slope is 3 and y-intercept is 4.
Solution We already know the slope-intercept form of the equation:
y = mx + c
The slope m is given as 3. The y-intercept c is given as 4. Nothing much to do here then. The required equation will be
y = 3x + 4
Here’s how the line looks.
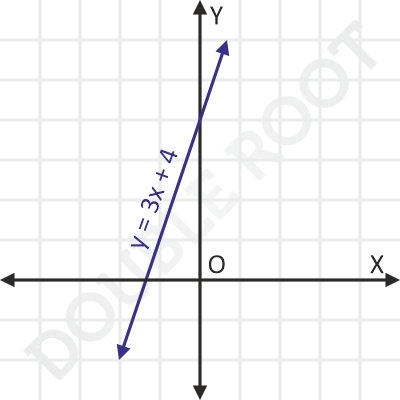
Example 2 Find the equation of the line which passes through a point on the Y-axis 5 units below the origin, and makes an angle of 45° with the X-axis.
Solution Here, “passes through a point on the Y-axis 5 units below the origin” is a fancy way to say that the y-intercept is -5.
And, “makes an angle of 45° with the X-axis” implies that the slope is tan45°, which equals 1.
By plugging in the values in the slope-intercept form, we’ll get the required equation as
y = 1x + (-5)
or
y = x – 5
Here’s how the line looks.
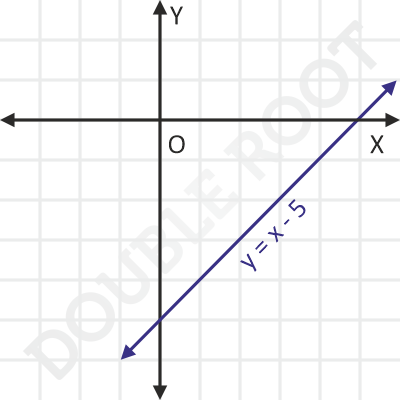
Example 3 A line passing through the origin has the slope as -2. Find its equation.
Solution Since the line passes through the origin, the y-intercept must be 0. Now, let’s use the slope-intercept form:
y = mx + c
On substituting the above values, we’ll get:
y = -2x + 0
or
y = -2x
Here’s how the line looks.
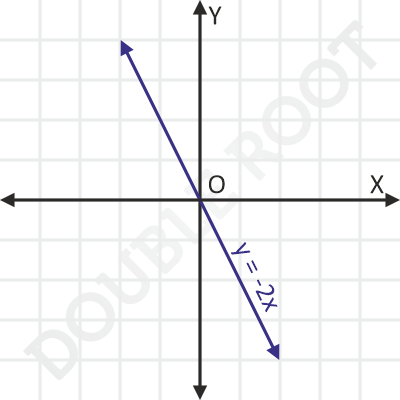
That’s all for this lesson. The next one will discuss another form of the equation of the line, known as the intercept form.