In this lesson, we’ll derive something known as the slope-intercept form of the equation of a line. Let’s see what it’s all about.
Suppose a line makes an angle θ with the X-axis, and passes through a point on the Y-axis, at a distance c from the origin.
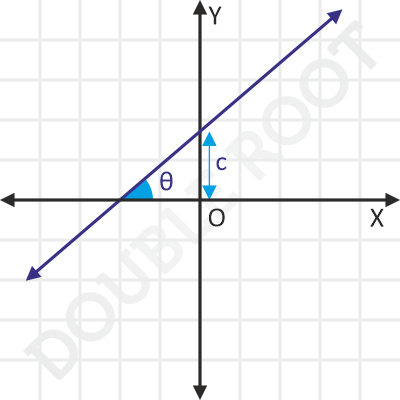
How can we find the equation of this line?
To do that, we’ll take any point P(x, y) on the line, and find a relation between its coordinates which will always hold true.
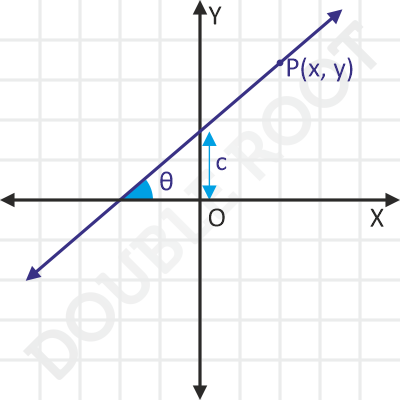
Let’s make some constructions in the previous figure.
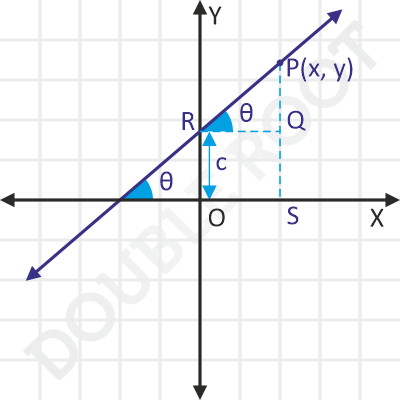
We constructed PS parallel to the Y axis, and RQ parallel to the X axis, where Q lies on PS.
Now,
PS = y
QS = RO = c
⇒ PQ = PS – QS = y – c
Also,
QR = x
Therefore, in ΔPQR, we have:
tanθ = PQ/QR
⇒ tanθ = (y – c)/x (substituting from above)
And, that’s it! We’ve found a relation that always holds true, regardless of the position of P(x, y).
We can rearrange the terms above to get:
y = (tanθ)x + c
We generally express this in the following form:
y = mx + c (where m = tanθ)
By the way, this c is called the y-intercept, which will be positive or negative depending upon whether the point (at which the line intersects the Y axis) lies above or below the origin. When the line passes through the origin, c equals zero.
And the tangent of the angle made by the line (i.e. tanθ) is known as the slope of the line. The convention is to measure the angle in the anticlockwise direction with the X-axis.
So, y = mx + c is the equation of a line whose slope is m and y-intercept is c. Hence the name – the slope-intercept form.
Before I end the lesson, let’s think about one thing – did we really need both θ and c? What if only θ was given? What if only c was given?
Let’s look at the case where only θ is given. The simulation below shows a line where θ = 45°. Is the line unique? Try dragging the red point.
As you would’ve noticed, a unique line won’t be possible here. This is because the line can be dragged parallely, keeping θ the same.
And here’s the situation if only c is given. The simulation below shows a line where c = 2. Is the line unique? Try dragging the red point.
Again, a unique line won’t be possible, as the line can freely rotated about that point on the Y axis, keeping c the same.
So, we’ll need both θ and c, for there to be a unique line. For example, if we fix θ as 45° and c as 2, then the line cannot be dragged or rotated, as doing so would change the values of θ and/or c. Hence, the line would be fixed or unique.
That’s about it for this lesson. Here’s a simulation where you can vary the slope and y-intercept of a line and observe how the line changes. Have fun!
Lesson Summary
- The equation of a line which makes an angle of θ (measured anticlockwise) with the X axis and has the y-intercept c is given by y = mx + c, where m = tanθ.
In this lesson, we took c to be positive, θ to be acute, and P(x, y) in the first quadrant and derived the equation of the line. Will we get the same equation if, say, c was negative or θ was obtuse, or P(x, y) was in some other quadrant?
In the next lesson, I’ll take different orientations of the line and different positions of P, and try deriving the equation. Let’s see what we get. Meet me there!