This lesson will cover a few examples related to the position of a point with respect to line.
Example 1 Show that the points (1, 2) and (3, 4) lie on the opposite sides of the line x + y – 4 = 0.
Solution To prove this, we’ll start by substituting the coordinates in the given equation, followed by comparing the signs of the two expressions we get.
On substituting (1, 2), we get:
1 + 2 – 4 or -1
On substituting (3, 4), we get:
3 + 4 – 4 or 3
Since we got expressions of opposite signs, the two points lie on the opposite side of the line. Here’s how things look:
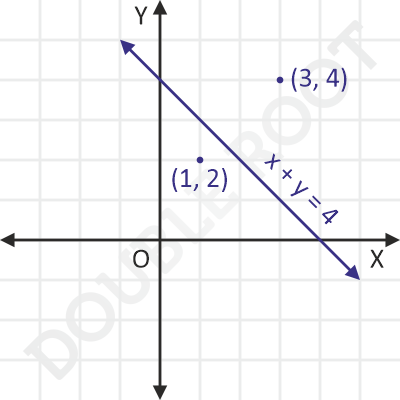
Example 2 Show that the points (0, 2) and (-3, -1) lie on the same sides of the line 2x – 3y = 6.
Solution Again, we’ll start by substituting the coordinates in the given equation. And this time, we should get two expressions of the same sign.
Before substituting, we’ll bring all terms the given equation to the LHS. We’ll get:
2x – 3y – 6 = 0
Now, on substituting (0, 2), we get:
2(0) – 3(2) – 6 or -12
On substituting (-3, -1), we get:
2(-3) -3(-1) – 6 or -9
Since we got expressions of the same signs, the two points lie on the same side of the line. Here’s a figure to illustrate:
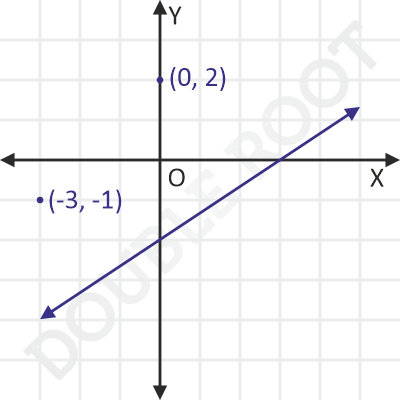
Example 3 Show that the point (1, 2) lies inside the triangle whose sides are along the lines x + y = 4, x – 2y = 1 and y – 2x = 4.
Solution This one requires a little more thinking. Let’s take a look at the three lines.
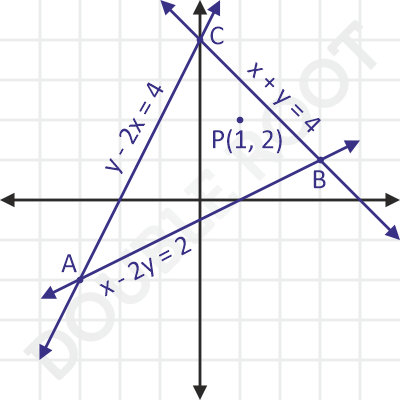
Now here’s the thing. If P is any point inside a triangle ABC, then
P and A will be on the same side of BC,
P and B will lie on the same side of CA, and
P and C will lie on the same side of AB.
Take a look at the above figure again to make sense of the these conditions. If either of these three is not met, then the point P will lie outside the triangle.
Now, to prove that P lies inside the triangle formed by the given lines, we’ll have to find the coordinates of the three vertices, and verify the above three conditions.
Let’s do that!
Side AB: x – 2y – 2 = 0
Sign with respect to C(0, 4): 0 – 2(4) – 2 = -10 (negative)
Sign with respect to P(1, 2): 1 – 2(2) – 2 = -7 (negative)
This means that P and C lie on the same side of AB.
Side BC: x + y – 4 = 0
Sign with respect to A(-3, -2): -3 – 2 – 4 = -9 (negative)
Sign with respect to P(1, 2): 1 + 2 – 4 = -1 (negative)
This means that P and A lie on the same side of BC.
Side CA: -2x + y – 4 = 0
Sign with respect to B(3, 1): -2(3) + 1 – 4 = -9 (negative)
Sign with respect to P(1, 2): -2(1) + 2 – 4 = -4 (negative)
This means that P and B lie on the same side of CA.
And that’s it! We got what we needed. Given the three conditions above, the point P must lie inside the triangle. Try solving the problem once again on your own.
That’s about enough for this topic. I’ll talk about family of lines in the next lesson. See you there!