7. Point Slope Form

This lesson will cover the point-slope form of the equation of a straight line.

Consider a line which passes through the point (x₁, y₁), and makes an angle θ with the X-axis.

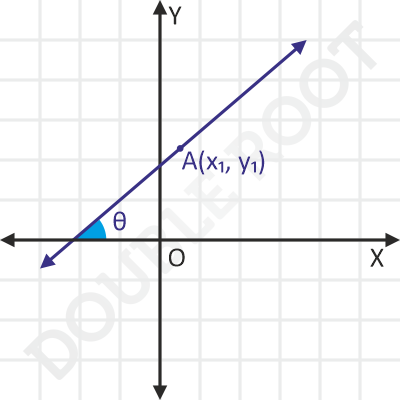
How can we find its equation? Let’s explore two different methods.

Method I

We’ll start with the slope-intercept form of the line. Let’s assume the equation to be y = mx + c (where m = tanθ).

Now, we do not know the value of c, but instead we know that the line passes through (x₁, y₁).

Recall that the equation of a line (or any curve in general) is a relation that holds true for every point on the line. Since the line passes through (x₁, y₁), the relation must hold true for this point as well.

Therefore, we can substitute these coordinates into the equation to get:

y₁ = mx₁ + c

On rearranging, this gives

c = y₁ – mx₁

We’ve got the value of c. Substituting this into the original equation, we’ll get the required equation as:

y = mx + y₁ – mx₁

The terms can be rearranged as follows:

y – y₁ = m(x – x₁)

And that’s the equation we were looking for – the point-slope form.

Method II

Here, we’ll derive the equation from scratch. Let’s take any point P(x, y) on the line.

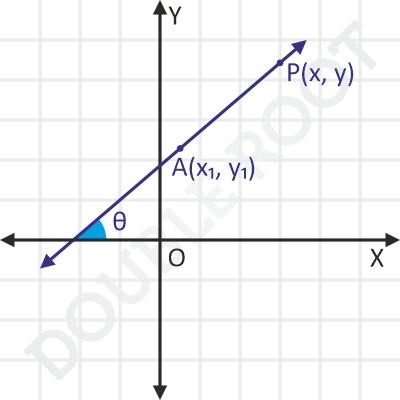
Now, let’s construct a couple of line segments.

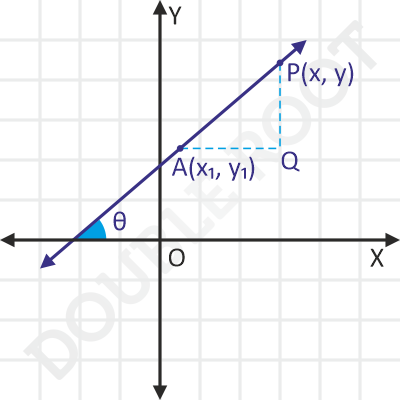
We constructed AQ parallel to the X-axis and PQ parallel to the Y-axis.

Now, in ΔAQP, we have:

tanθ = PQ/AQ

This means that:

tanθ = (y – y₁)/(x – x₁)

On rearranging the terms, we get

y – y₁ = tanθ(x – x₁)

⇒ y – y₁ = m(x – x₁)

Same equation as earlier.

Lesson Summary

1. The equation of a line having a given slope m, and passing through a given point (x₁, y₁) is given by y – y₁ = m(x – x₁)

This form will be one of the most frequently used forms, so keep it handy. See you in the next lesson with some examples!