Hello. In this lesson, I’ll cover a few examples related to angle bisectors of a pair of straight lines.
Example 1 Find the equation of angle bisectors for the pair of lines 3x + 4y – 7 = 0 and 4x + 3y – 7 = 0.
Solution Here, we can directly apply the formula that we derived previously.
The required equations are:
\(\frac{3x+4y-7}{\sqrt{{3}^2 + {4}^2}}=\pm\frac{4x+3y-7}{\sqrt{{4}^2 + {3}^2}}\)
On rearranging the terms, we get two bisectors as:
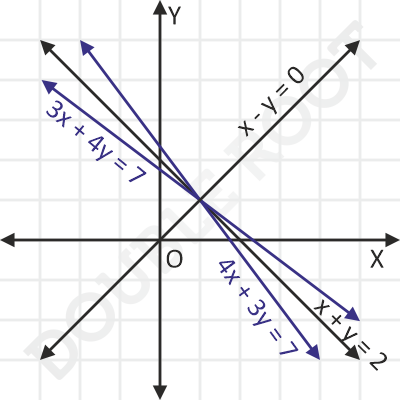
x – y = 0
x + y = 2
Note that the two bisectors are perpendicular (and will always be).
We can find some more information about these bisectors. By finding out the angle between any one of the bisectors and one of the lines, we can distinguish between the acute and obtuse angle bisector.
Let’s try that out. We’ll find the angle θ between the bisector x – y = 0, and one of the lines, 3x + 4y – 7 = 0. Using the formula for the angle between two lines, we’ll get:
|tanθ| = 7
Since |tanθ| is greater than 1, θ must be greater than 45°. This means that x – y = 0 the bisector of the obtuse angle between the given lines.
Here’s how things look.
Example 2 Find the equations of the lines which are equally inclined to the lines 5x + 12y + 7 = 0 and 12x – 5y – 17 = 0, and pass through the point (1, 2).
Solution Here are the two lines.
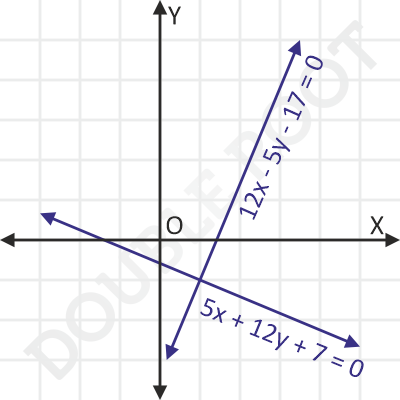
And these are the lines we’re looking for.
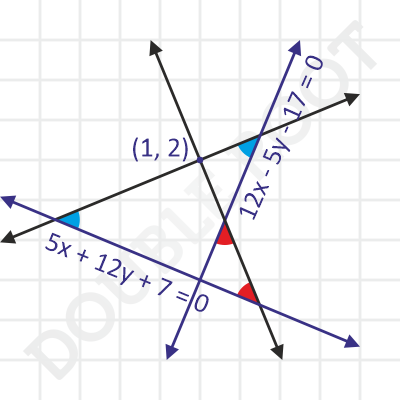
The black lines are both equally inclined to the given lines, and pass through (1, 2) as well.
There are (atleast) two methods to solve this problem – similar to the ones we discussed in the previous lesson. I’ll skip the first one here, but do try solving the problem using that method.
The other method involves angle bisectors. Now, angle bisectors themselves are equally inclined to the two given lines. That means, the required lines will be parallel to these angle bisectors.
So, if we get the slope of the angle bisectors, we can use the point-slope form to find the equation of the required lines.
To find the slopes, we’ll first find the equations of these angle bisectors. These are:
\(\frac{5x+12y+7}{\sqrt{{5}^2 + {12}^2}}=\pm\frac{12x-5y-17}{\sqrt{{12}^2 + {5}^2}}\)
And, these equations simplify to:
17x + 7y – 10 = 0
7x – 17y – 24 = 0
The slopes of these bisectors are -17/7 and 7/17 respectively. And, the required lines will have the same respective slopes, since they’re parallel to these bisectors.
Finally, the required equations will be:
y – 2 = (-17/7)(x – 1) or 17x + 7y – 31 = 0
y – 2 = (7/17)(x – 3) or 7x – 17y + 27 = 0
And, that’s all for this lesson!
I’ll return to straight lines sometime later with Pair of Straight Lines. Till then, you can head back to the lessons page to find something else to learn.