17. Angle Between Two Lines

This lesson will focus on finding the angle between two given lines, given their slopes (or equations).

Suppose we have two lines whose slopes are known, say m₁ and m₂. Then, how can we find the angle between these lines?

In other words, given the angles made by the lines with the X-axis, how can we find the angle made by the lines with each other?

Take a look at the figure below. Can we find θ in terms of θ₁ and θ₂?

Turns out it’s pretty simple to do that. Using the exterior angle property in the triangle above, we have:

θ = θ₁ – θ₂

Then,

tanθ = tan(θ₁ – θ₂)

⇒ tanθ = (tanθ₁ – tanθ₂)/(1 + tanθ₁tanθ₂)

Now, on replacing tanθ₁ and tanθ₂ by m₁ and m₂ respectively, we get:

tanθ = (m₁ – m₂)/(1 + m₁m₂)

And, we’re done. We related the angle between the two lines (θ) with the slope. That is, given the slopes, we can get the value of tanθ (and hence θ).

A small note. The expression on the RHS will change its sign depending upon whether we take m₁ as the slope of the first line or the second.

For example, if m₁ = 1/2 and m₂ = -1/3, then tanθ comes out to be 1, which means θ = 45°. But if we take m₁ as -1/3 and m₂ as 1/2, then tanθ would come out to be -1, which means θ = 135°.

Geometrically, the two different angles represent the same situtation with respect to the lines. Take a look.

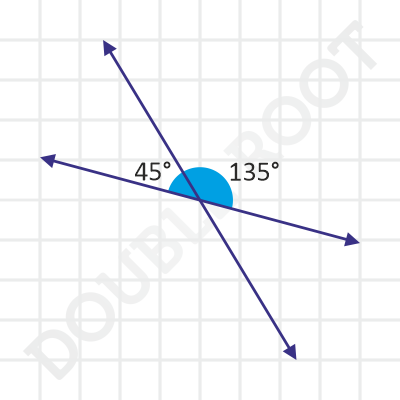
So, we needn’t worry about this issue much. But to keep things clean, we use a modulus sign on the previous expression (on the RHS).

This will always give a positive value of tanθ (i.e., θ will always be an acute angle). The final formula looks something like this:

tanθ = |(m₁ – m₂)/(1 + m₁m₂)|

Lesson Summary

1. The angle (θ) between two lines, whose slopes are m₁ and m₂ is given by tanθ = |(m₁ – m₂)/(1 + m₁m₂)|.

In the next lesson, I’ll talk about the relation between the slopes of parallel and perpendicular lines.