This lesson will cover a few examples involving angle between lines.
Let’s start with a simple one.
Example 1 Find the angle between
(i) the lines 2x + y – 4 = 0 and 3x – y + 1 = 0.
(ii) the lines AB and CD, where A ≡ (1, 2), B ≡ (3, 4), C ≡ (6, 7), and D ≡ (8, 5).
Solution In both parts, we’ll first find the slopes of the two lines, say m1 and m2. Then we’ll apply the formula that we derived in the previous lesson:
tanθ = |(m1 – m2)/(1 + m1m2)|
(i) Using the expression we derived here, the slope of the first line is given by:
m1 = -(2)/1 = -2
Simliarly, the second line’s slope is given by:
m2 = -(3)/(-1) = 3
Now, we can apply the above formula:
tanθ = |(-2 – 3)/(1 + (-2)(3))|
⇒ tanθ = 1
⇒ θ = 45°
Note that in case we didn’t use the absolute value, we could have obtained tanθ = -1 or θ = 135°. It doesn’t make any difference though. The two different angles correspond to the same situation geometrically (as I’ve explained previously). Here’s a figure to illustrate.
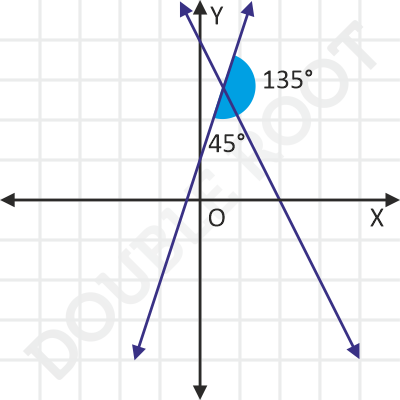
(ii) This is similar to the previous one. Except that, the equations aren’t given (which we do not require anyway).
Instead, we’ve been provided with points, through which we can calculate the slopes of the lines joining them.
Now, the slope of AB equals:
(4 – 2)/(3 – 1) or 1
And, the slope of CD equals
(5 – 7)/(8 – 6) or -1
If we apply the prevous formula, we’ll get:
tanθ = |(1 – (-1))/(1 + (-1)(1))|
⇒ tanθ = 2/0
Since the denominator is zero, it means that tanθ is undefined. That means θ = 90°, i.e., the lines are perpendicular.
We could also have figured out the same by multiplying the slopes. Since the product is -1, the lines must be perpendicular (explanation here).
Example 2 Find the equation of the line which passes through the point (1, 2) and is
(i) parallel to the linr x + 2y – 3 = 0.
(ii) perpendicular to the line 3x + y = 4.
Solution For both parts, I’ll discuss two different methods.
(i) Method I
Since the lines are parallel, the slope of the required line would the same as that of the given line, which equals:
–(coefficient of x)/(coefficient of y)
= -1/2
Now, to find the equation, we can use the point-slope form. We’ll get:
y – 2 = (-1/2)(x – 1)
This simplifies to:
x + 2y – 5 = 0
Method II
Let’s take a look at the answer obtained in the previous method.
x + 2y – 5 = 0
If we compare with the one given (i.e. x + 2y – 3 = 0), we can see that the coeffiecients of x and y are the same, and only the constants are different.
So, we can directly assume the required line to be:
x + 2y + k = 0
This works because any line of the form x + 2y + k = 0 has the slope -1/2, which is the same as that of the given line.
And therefore, x + 2y + k is always parallel to x + 2y – 3 = 0. Keep changing k, and you’ll keep getting different lines, but each of them would be parallel to the given line.
But of these many parallel lines, we only need the particular one that passes through (1, 2). In other words, (1, 2) must satisfy its coordinates. We’ll get:
1 + 2(2) + k = 0
⇒ k = -5
Therefore, the required equation is:
x + 2y – 5 = 0
So, remember that any line parallel to a given line ax + by + c = 0 would be:
ax + by + k = 0
The two lines have the same slope (-a/b), which means that they’re parallel.
(ii) Method I
Since the two lines are perpendicular, the product of the slopes of the required line and the given line must equal -1.
If we assume the slope of the required line to be m, we have:
m x (-3) = -1 or m = 1/3
Using the point-slope form again, we get the required equation as:
y – 2 = 1/3(x -1)
This simplifies to:
x – 3y + 5 = 0
Method II
Let’s take a look at the answer obtained:
x – 3y + 5 = 0
If we compare this with the given line (i.e. 3x + y – 4 = 0), we can see that the coefficients of x and y have interchanged and the sign of one of these coefficients is reversed.
So, we can directly assume the required line to be:
x – 3y + k = 0
This works because any line of the form x – 3y + k = 0 has the slope 1/3, the product of which with the slope of the given line equals -1.
And therefore, x – 3y + k is always perpendicular to 3x + y – 4 = 0. Keep changing k, and you’ll keep getting different lines, but each of them would be perpendicular to the given line.
But of these many perpendicular lines, we only need the particular one that passes through (1, 2). In other words, (1, 2) must satisfy its coordinates. We’ll get:
1 – 3(2) + k = 0
⇒ k = 5
Therefore, the required equation is:
x – 3y + 5 = 0
So, remember that any line perpendicular to a given line ax + by + c = 0 would be:
bx – ay + k = 0
The two lines have the slopes -a/b and b/a, the product of which equals -1, meaning that they’re perpendicular.
Lesson Summary
- Any line parallel to a given line ax + by + c = 0, will be of the form ax + by + k = 0.
- Any line parallel to a given line ax + by + c = 0, will be of the form bx – ay + k = 0.
- The value of k will be obtained by some additional given condition in both cases above.
That’s all for this lesson. In the next one, I’ll continue with a few more examples on the same concept.