Welcome to part 2 of the previous lesson ! I’ll take up a few more examples. Let’s go!
Two fixed points are given, say A and B. What will be the locus of the moving point P such that the sum of its distances from A and B should always be equal to AB. That is, PA + PB = AB.
Hmm.. this looks familiar. If you go back here, and recall that this was the condition for three points to be collinear.
Well, that tells me that A,P and B must be collinear (atleast). What about the locus? A figure to the rescue !
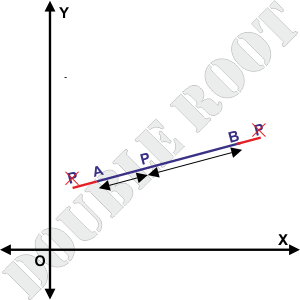
For any point P on AB, PA + PB = AB. So the locus of P will be the line segment AB. Note that P cannot go beyond A or B, because towards the left of A, PB > AB, so the sum PA + PB can never be equal to AB. Same is the case for going beyond B.
A similar example. How about the condition PA – PB = AB? I suggest you try it on your own before reading further.
The condition can be written as PA = PB + AB, which tells us that A, B, and P are collinear. The difference here is that B should lie between A and P, unlike the previous case.
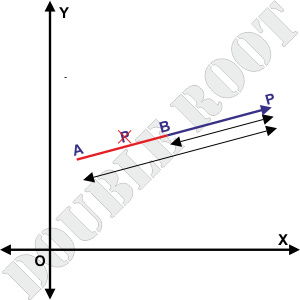
So the locus will be a ray originating from B, opposite to the direction of A and parallel to AB. Similarly, if we put the condition as PB – PA = AB, the locus will be a ray originating from A, away from B and parallel to AB.
Last example, plus a homework ! Suppose there is a point P, moving in a plane, such that APB is always a right angle, where A and B are two fixed points. What will be the locus of P?
This isn’t very easy to imagine. But you’ve done something related to this in class 10th maybe. Hmm… lets draw something.
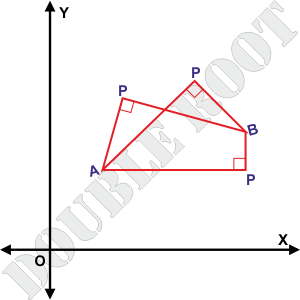
Angle APB is always 90o. That reminds me of something, “Angle in a semicricle is always 90o“.
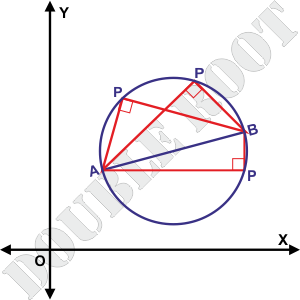
So the locus of P will be a circle whose diameter is AB.
That’s it for the introduction part ! Hope you now understand what locus means. Don’t worry if you’re still confused. Most people are. I suggest you go through the lesson once again.
In the next lesson, I’ll cover equation of the locus, or in general what an equation (of a curve) means in coordinate geometry. See you there !
Oh.. and the homework ! In the last example, what will be the locus of P if the angle should be always 60 degrees instead of 90?