This lesson will cover a few examples, illustrating equations of tangents to circles, and their points of contacts.
Example 1 Find the equation of the tangent(s) of slope 4/3 to the circle x2 + y2 = 25. Also find the point(s) of contact.
Solution This problem is a direct application of the slope form of the tangent: \( y = mx ± a \sqrt{1+m^2} \) . The required equation is \(y = 4x/3 ± 5 \sqrt{1+(4/3)^2} \) , or 3y = 4x ± 25.
To find the points of contact, we’ll use the coordinates derived in the previous lesson: (–a2m/c, a2/c). The required points are (4, -3) and (-4, 3) (Here m = 4/3 and c = ± 25/3). Have a look at the following figure for clarity
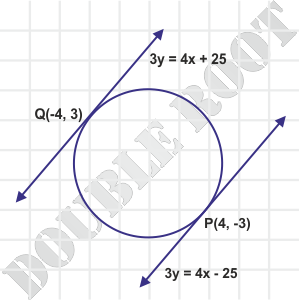
Example 2 Find the equation of the tangent(s) of slope 4/3 to the circle x2 + y2 – 4x – 6y + 12 = 0. Also find the point(s) of contact.
Solution Another problem involving direct application of the formula. But in this case, the center of the circle is not at the origin. We’ll use the second equation: \( (y + f) = m(x + g) ± r\sqrt{1+m^2} \)
The required equation is \( (y – 3) = 4(x – 2)/3 ± 1 \sqrt{1+(4/3)^2} \) . Or, 4x – 3y + 6 = 0 and 4x – 3y – 4 = 0.
To find the points of contact, we’ll apply the formula again. I’ll skip the calculations. The required points are (6/5, 18/5) and (14/5, 12/5).
Psst! There’s another way to find the point of contact – it will be the foot of perpendicular from the center to the tangent.
Example 3 Find the value(s) of c for which y = 2x + c is a tangent to the circle x2 + y2 = 1
Solution We’ve already done a similar problem earlier, using condition of tangency. Here, I’ll show a different approach.
We know that the line y = mx + c will be a tangent to the circle x2 + y2 = a2 when c = ±a\( \sqrt{1+m^2} \) . Here a = 1, and m = 2, therefore c = ±1\( \sqrt{1+2^2} \) or ± \( \sqrt{5} \).
Example 4 Find the value(s) of m for which 3x + 4y = c is a tangent to the circle x2 + y2 – 4x – 6y – 12 = 0.
Solution Again, I’ll use the formula instead of condition of tangency, just to illustrate. Any tangent to the given circle looks like \( (y – 3) = m(x – 2) ± 5 \sqrt{1+m^2} \) or \( y = mx + 3 – 2m ± 1 \sqrt{1+m^2} \) .
The slope of the given line is –3/4. So all we have to plug in this value in the slope form of the tangent. We get y = –3x/4 + 3 – 2(–3/4) ± 5\( \sqrt{1+(3/4)^2} \) or 3x + 4y = 43, and 3x + 4y = –7. Therefore, c = 43 or – 7.
That’ll be all for this lesson. Next, I’ll be talking about tangents at a point. See you there!