Example 1 Find the equation of the circle, whose diameter’s end points are (1, 3) and (4, 2).
Solution Pretty simple. Using the form we derived in the previous lesson, the required equation is (x – 1)(x – 4) + (y – 3)(y – 2) = 0, or x2 + y2 – 5x – 5y + 10 = 0.
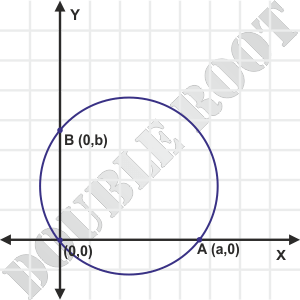
Example 2 Find the equation of the circle passing through the points (0, 0), (a, 0) and (0, b).
Solution I’ve covered a similar example in a previous lesson (see Example 9). But now we have a better method to obtain the equation.
Observe that the points (a, 0) and (0, b) lie on the X and the Y axes respectively. That means angle AOB = 90°, which implies that AB must be the diameter of the circle.
Therefore the required equation is (x – a)(x – 0) + (y – 0)(y – b) = 0, or x2 + y2 – ax – by = 0.
Note that the center of the circle is (a/2, b/2) (midpoint of AB, the diameter), and the radius is \( \sqrt{a^2+b^2}/2 \) (half of AB, the diameter)
Example 3 Find the equation of the circle, whose chord with end-points as (1, 2) and (0, 4) subtends an angle of 45° at its circumference.
Solution This equation type was also covered in the previous lesson.
I’ll use that equation directly: (x – 1)(x – 0) + (y – 2)(y – 4) = ± cot45° [(y – 2)(x – 0) – (y – 4)(x – 1)].
As I mentioned earlier, there’ll be two different circles satisfying the given conditions: x2 + y2 – 3x – 7y + 12 = 0 and x2 + y2 + x – 5y + 4 = 0.
And that’s it for this lesson. See you in the next with the parametric equation of the circle.