This lesson will cover a few more examples on equations of circles.
Example 6 Find the centre and the radius of the circle x2 + y2 – 4x + 6y – 3 = 0.
Solution Comparing this with the general equation of the circle, i.e. x2 + y2 + 2gx + 2fy + c = 0, we have g = –2, f = 3 and c = – 3.
The centre will be (–g, –f) or (2, –3), and the radius will be \( \sqrt{g^2+f^2 – c} = \sqrt{2^2+{(-3)}^2 – (-3)} \) = 4
Example 7 Find the equation of the circle which touches the line 3x + 4y = 5 and has centre (2, 1).
Solution We’ve been given the centre of the circle, but not the radius. Instead, we know that the circle touches a line.
What does that tell us? Recall, from your earlier classes, that the radius of a circle is perpendicular to the tangent. In other words, the perpendicular distance from the centre to a tangent will be equal to the radius.
To find the radius, all we have to do is find the perpendicular distance of the line from the given point (i.e. the centre). Using the formula we derived earlier (distance of a point from a line), the required distance is |3(2) + 4(1) – 5|/\( \sqrt{3^2 + 4^2} \) = 1.
Therefore the required equation is (x – 2)2 + (y – 1)2 = 1

Example 8 Find the equation of circle lying in the first quadrant, which touches both the axes and has radius 3.
Solution This one’s slightly different. We’ve been given the radius of the circle, but not the centre. Instead, we know that the circle touches both the axes, and lies in the first quadrant. This is what the circle would look like.
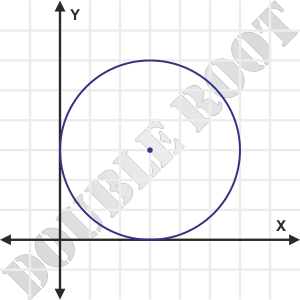
Since the circle touches the axes, the distance of the centre from both the axes must be equal to the radius (something similar to the previous example). And, the distances from the axes are nothing but the coordinates of the centre.
Therefore the coordinates of the centre must be (3, 3). And, the required equation will be (x – 3)2 + (y – 3)2 = 32
We have a special equation – the equation of a circle touching both the axes and lying in the first (or the third) quadrant, whose radius is ‘a’, will be (x – a)2 + (y – a)2 = a2
(Also find the equation of those which lie in the 2nd or the 4th quadrant)
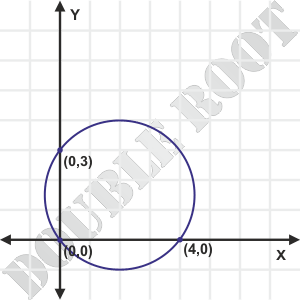
Example 9 Find the equation of the circle which passes through the points (0, 0), (4, 0) and (0, 3).
Solution We’ll have better and interesting ways to find equations of circles passing through three points, but I’ll cover a basic method for now.
We’ll start with the general equation, which has three unknowns: g, f and c. The values of these unknowns will be found out by forming three equations in these unknowns, obtained by substituting the given coordinates in the circle’s equation. Let’s begin.
Let the equation of the circle be x2 + y2 + 2gx + 2fy + c = 0.
On substituting (0, 0), we get (0)2 + (0)2 + 2g(0) + 2f(0) + c = 0
On substituting (4, 0), we get (4)2 + (0)2 + 2g(4) + 2f(0) + c = 0
Finally, on substituting (0, 0), we get (0)2 + (3)2 + 2g(0) + 2f(3) + c = 0
On solving the equations, the values of g, f and c are obtained as -2, -3/2 and 0 respectively.
The required equation is x2 + y2 – 4x – 3y = 0.
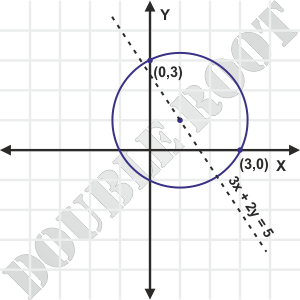
Example 10 Find the equation of the circle which passes through the points (0, 3), (3, 0) and whose centre lies on the line 3x + 2y = 5
Solution This one is somewhat similar to the previous problem. We’ll again assume the equation to be x2 + y2 + 2gx + 2fy + c = 0, and obtain two equations by substituting the given points.
On substituting (3, 0), we get (3)2 + (0)2 + 2g(3) + 2f(0) + c = 0 and on substituting (0, 3), we get (0)2 + (3)2 + 2g(0) + 2f(3) + c = 0. What about the third?
It has been given that the centre, i.e. (-g, -f) lies on the line 3x + 2y = 5. This will give us the third equation, which is 3(-g) + 2(-f) = 5.
On solving the equations, the values of g, f and c are obtained as -1, -1 and -3 respectively.
The required equation is x2 + y2 – 2x – 2y – 3 = 0.
I’ll cover a couple of more equations of a circle in the next lesson. See you there!