This lesson will cover a few solved examples about what I talked about in the previous lesson.
Example 1 Find the equation of the circle whose centre is at the origin and whose radius is 4.
Solution This is a simple one. We’ll use the standard equation of the circle straight away.
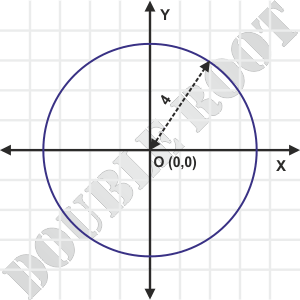
The required equation is x2 + y2 = 42.
Example 2 Find the equation of the circle whose centre is at the origin and which passes through the point (3, 2).
Solution A slight twist in the previous example. We know that the standard equation is
x2 + y2 = r2
The radius (r) hasn’t been given. But it is known that the circle passes through the point (3, 2), which means its coordinates must satisfy the circle’s equation.

By substituting the coordinates in the given equation, we have
32 + 22 = r2
⇒ r2 = 13
Therefore, the required equation is x2 + y2 = 13.
Example 3 Find the equation of the circle whose centre is at (1, 4) and radius equals 3.
Solution We can’t use the standard equation in this case. We’ll the second variant of the equation, which I talked about in the previous lesson, i.e. (x – x1)2 + (y – y1)2 = r2.
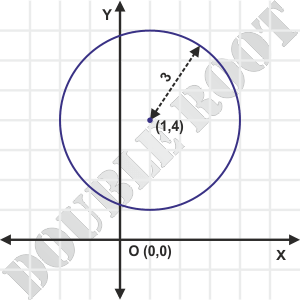
The required equation will be (x – 1)2 + (y – 4)2 = 32.
Example 4 Find the equation of the circle whose centre is at (3, 4) and passes through the point (2, 6).
Solution This problem is somewhat similar to the second example above. In this case, the centre is given but not the radius. Instead, it is known that the circle passes through a given point.
To begin, the equation of the circle is
(x – 3)2 + (y – 4)2 = r2
Next, we’ll substitute the given coordinates in the equation to obtain the value of r. We’ll get
(2 – 3)2 + (6 – 4)2 = r2
⇒ r2 = 5
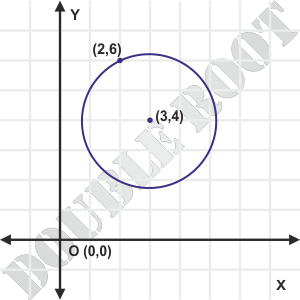
Therefore, the required equation is (x – 3)2 + (y – 4)2 = 5.
Example 5 Find the equation of the circle whose center is the point of intersection of the lines x + 2y = 4 and x + y = 1, and whose radius equals 2.
Solution In this case, the coordinates of the center will be obtained by solving the given equations of the lines: (-2, 3).
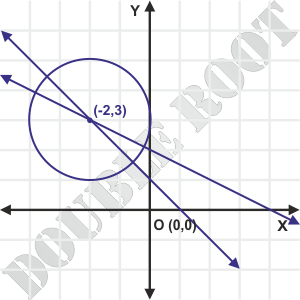
The required equation is (x – 2)2 + (y + 1)2 = 22.
That’s all for this lesson. The next one will cover a few more examples related to equations of the circle.